Convolution Theorem Laplace Transform Examples

In mathematics the Laplace transform named after its discoverer Pierre-Simon Laplace l ə ˈ p l ɑː s is an integral transform that converts a function of a real variable usually in the time domain to a function of a complex variable in the complex frequency domain also known as s-domain or s-planeThe transform has many applications in science and engineering because. We also derive the formulas for taking the Laplace transform of functions which involve Heaviside functions.

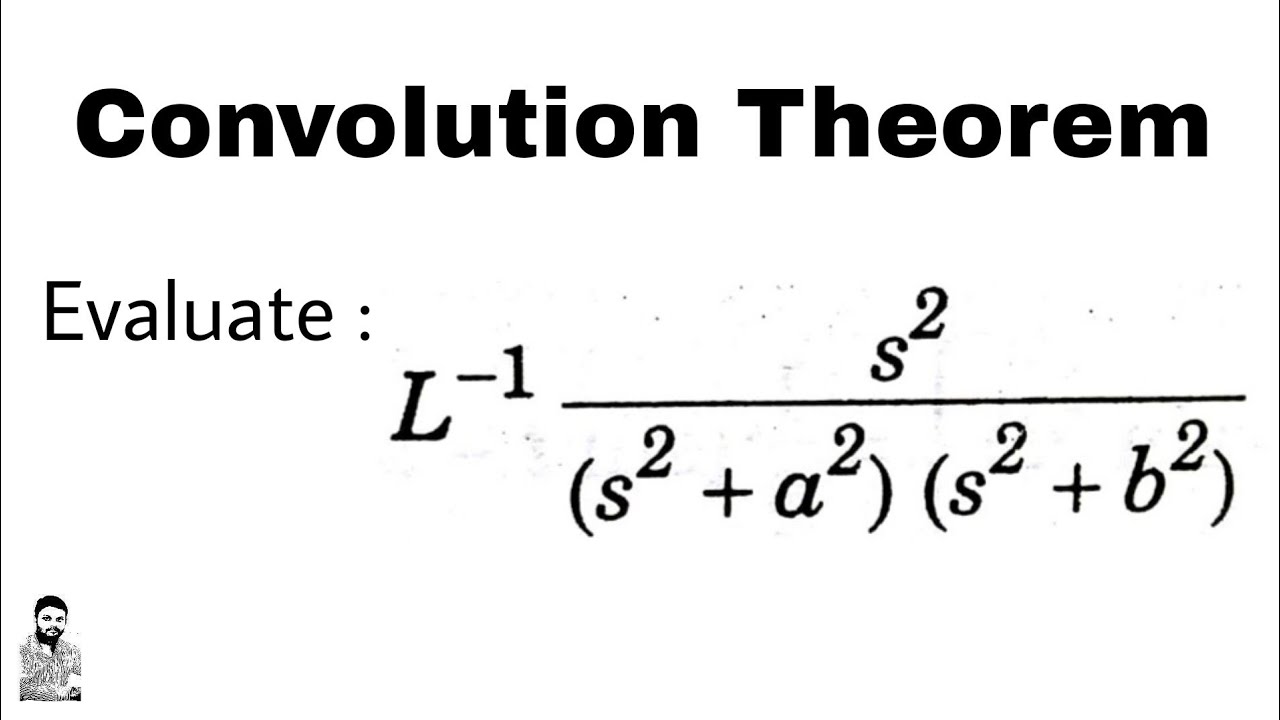
20 Convolution Theorem Problem 2 Inverse Laplace Transforms Youtube

We can use a convolution integral to do this.

. The unique capability of graphs enables capturing the structural relations among data and thus allows to harvest more insights compared to analyzing data in isolation. Here is a set of videos that explains it and shows several examples. Section 94 Geometric evaluation of the Fourier transform from the pole-zero plot pp.

Moreover Cauchy in 1816 and independently Poisson in 1815 gave a derivation of the Fourier integral theorem by means of an argument involving what we would now recognise as a sampling operation of the type associated with a delta function. We would like a way to take the inverse transform of such a transform. Here is a set of notes used by Paul Dawkins to teach his Differential Equations course at Lamar University.

The interval at which the DTFT is sampled is the reciprocal of the duration of the input sequence. Blackpenredpen - Proof of the Convolution Theorem. While the symbol t is used above it need not represent the time domain.

Mathematically it has the form. Then is called inverse Laplace Transform of. The convolution of f and g is written fg denoting the operator with the symbol.

Ft gt be the functions of time t then First shifting Theorem. Linear Systems Analysis inverse transform Additive property First shift theorem The Convolution Theorem. This section describes the applications of Laplace Transform.

Xs 1 s 1 s2 4. We again work a variety of examples illustrating how to use the table of Laplace transforms to do this as well as some of the manipulation of the given Laplace transform that is needed in order to use the table. Z-Transform An fiIflpreceding an acronym indicates fiInverseflas in IDTFT and IDFT.

Solving IVPs with Laplace Transforms - In this section we will examine how to use Laplace transforms to solve IVPs. There are many inverse Laplace transform online examples available for determining the inverse transform. The convergence criteria of the Fourier transform namely that the function be absolutely integrable on the real line are quite severe due to the lack of the exponential decay term as seen in the Laplace transform and it means that functions like polynomials exponentials and trigonometric functions all do not have Fourier transforms in the.

Find the inverse transform. 1 The inverse transform L1 is a linear operator. 22 Section 100.

The examples in this section are restricted to differential equations that could be solved without using Laplace transform. The inverse Laplace transform operates in a reverse way. Fourier optics is the study of classical optics using Fourier transforms FTs in which the waveform being considered is regarded as made up of a combination or superposition of plane wavesIt has some parallels to the HuygensFresnel principle in which the wavefront is regarded as being made up of a combination of spherical wavefronts also called phasefronts whose.

An equivalent definition is see commutativity. The main properties of Laplace Transform can be summarized as follows. That is to invert the transformed expression of Fs in Equation 61 to its original function ft.

Where s is the parameter of the Laplace transform and Fs is the expression of the Laplace transform of function ftwith 0 t. In mathematics the Dirac delta distribution δ distribution also known as the unit impulse is a generalized function or distribution over the real numbers whose value is zero everywhere except at zero and whose integral over the entire real line is equal to one. The current understanding of the impulse is as a linear functional that maps every continuous function to its value at zero.

Section 97 Analysis and characterization of LTI systems using the Laplace transform pp. On occasion we will run across transforms of the form Hleft s right Fleft s rightGleft s right that cant be dealt with easily using partial fractions. All of these concepts should be familiar to the student except the DFT and ZT which we will dene and study in detail.

Visit BYJUS to learn the definition properties inverse Laplace transforms and examples. Where called Besselsfunction. Learn about Laplace Transform Convolution 10 complete solutions to practice problems SVC.

I Solution decomposition theorem. Section 95 Properties of the Laplace transform pp. I Properties of convolutions.

The time function ft is obtained back from the Laplace transform by a process called inverse Laplace transformation and denoted by -1. Laplace transform is the integral transform of the given derivative function with real variable t to convert into a complex function with variable s. I Convolution of two functions.

I Impulse response solution. The advantage of starting out with this type of differential equation is that the work tends to be not as involved and we can always check our answers if we wish to. Laplace Transform Table 1 Laplace Transform Table 2.

Section 412 First-order and second-order systems pp. From the source of Science Direct. However it is often very challenging to solve the learning problems on graphs.

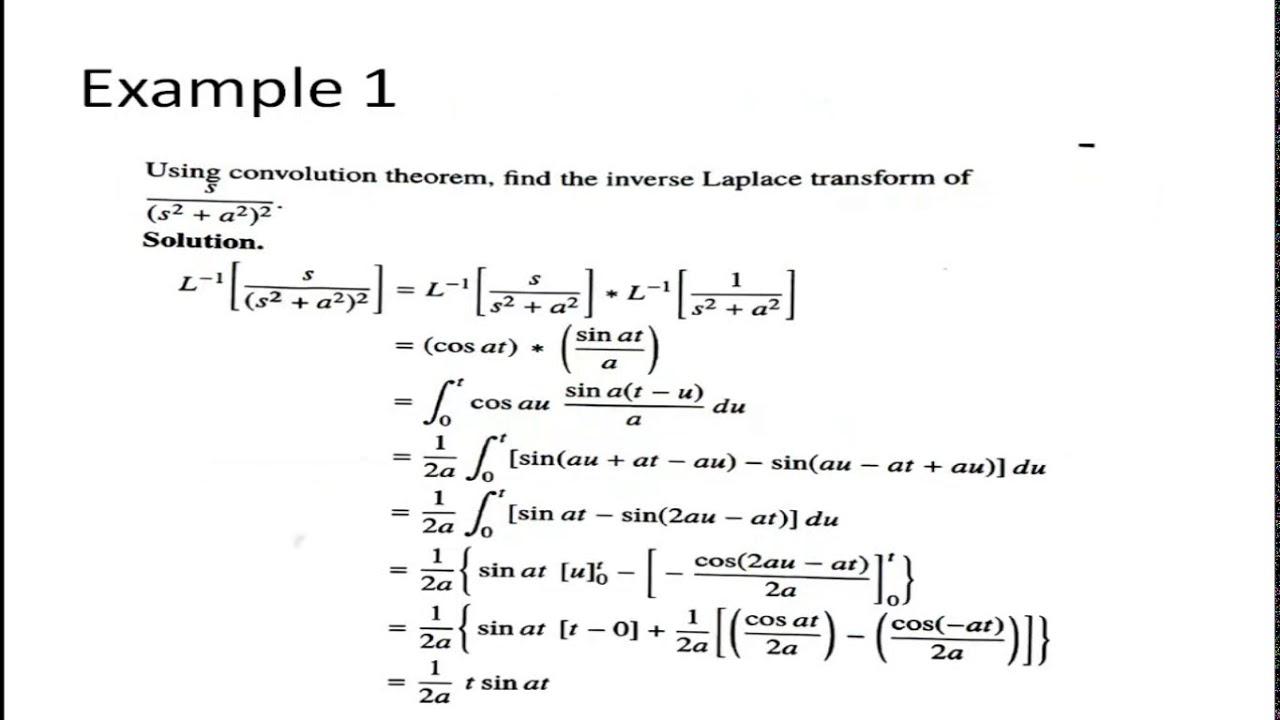
Inverse Laplace Transform by Convolution Theorem. Then 2 Applications of Laplace Transform in Science and Engineering fields. As such it is a particular kind of integral transform.

A table containing information about Laplace transforms is always available to the engineer. If you want to use the convolution theorem write Xs as a product. It is defined as the integral of the product of the two functions after one is reversed and shifted.

Section 4-9. The Inverse Laplace Transform 1. From the following table we will learn about the Laplace transform of various common functions.

Theorem Properties For every piecewise continuous functions f g and h hold. Graphical Concept of Convolution Videos. In this section we ask the opposite question from the previous section.

In mathematics the discrete Fourier transform DFT converts a finite sequence of equally-spaced samples of a function into a same-length sequence of equally-spaced samples of the discrete-time Fourier transform DTFT which is a complex-valued function of frequency. Discrete Fourier Transform ZT. In this section we will examine how to use Laplace transforms to solve IVPs.

In other words given a Laplace transform what function did we originally have. Get Widget With Customization. Let C 1 C 2 be constants.

And there are similar examples of the use of what are essentially delta functions by Kirchoff Helmholtz and of course Heaviside himself. 2 Review of the DT Fourier Transform 21 Denition and Properties. A Fourier transform FT is a mathematical transform that decomposes functions depending on space or time into functions depending on spatial frequency or temporal frequencyThat process is also called analysisAn example application would be decomposing the waveform of a musical chord into terms of the intensity of its constituent pitchesThe term Fourier transform refers to.

Laplace Transform of Bessels function. Below is an example of such a table. The examples in this section are restricted to differential equations that could be solved without using Laplace.

I Laplace Transform of a convolution. Graphs naturally appear in numerous application domains ranging from social analysis bioinformatics to computer vision. Included are most of the standard topics in 1st and 2nd order differential equations Laplace transforms systems of differential eqauations series solutions as well as a brief introduction to boundary value problems Fourier series and partial differntial.

If Lft Fs then the inverse Laplace transform of Fs is L1Fs ft.

Csir Ugc Net Convolution Theorem In Laplace Transform Part 1 Offered By Unacademy

Using The Convolution Theorem To Find The Inverse Laplace Transform Youtube

B Tech S2 S4 Laplace Transforms Convolution Theorem Youtube

Convolution Theorem Laplace Transforms Example Problem 1 Youtube